Many sea kayakers will be familiar with the 50/90 rule and the rule of thirds. If you're not, perhaps look at the relevant page in my navigation book? Briefly:
50/90 rule: flow attains 50% of max speed after 1 hour, 90% after 2 hours, 100% at 3 hours
Rule of thirds: the average flow is 1/3 of max speed in the first hour of the tide, 2/3 in the second hour, 3/3 in the third hour
Where do these rules come from? And do they make accurate predictions?
Approximating a sine wave
Both rules make the assumption that the flow speed follows a sine wave with 6 hours between times of slack water. The rules aren't strictly limited to situations where the time between slack waters is 6 hours - it's possible to split any tidal cycle into 6 equal periods and apply the rules.
We'll come back to whether the sine wave assumption is valid, but let's begin by considering whether the approximations to the sine wave are sensible. The plot below shows the estimates overlaid on the 6 hour sine wave:
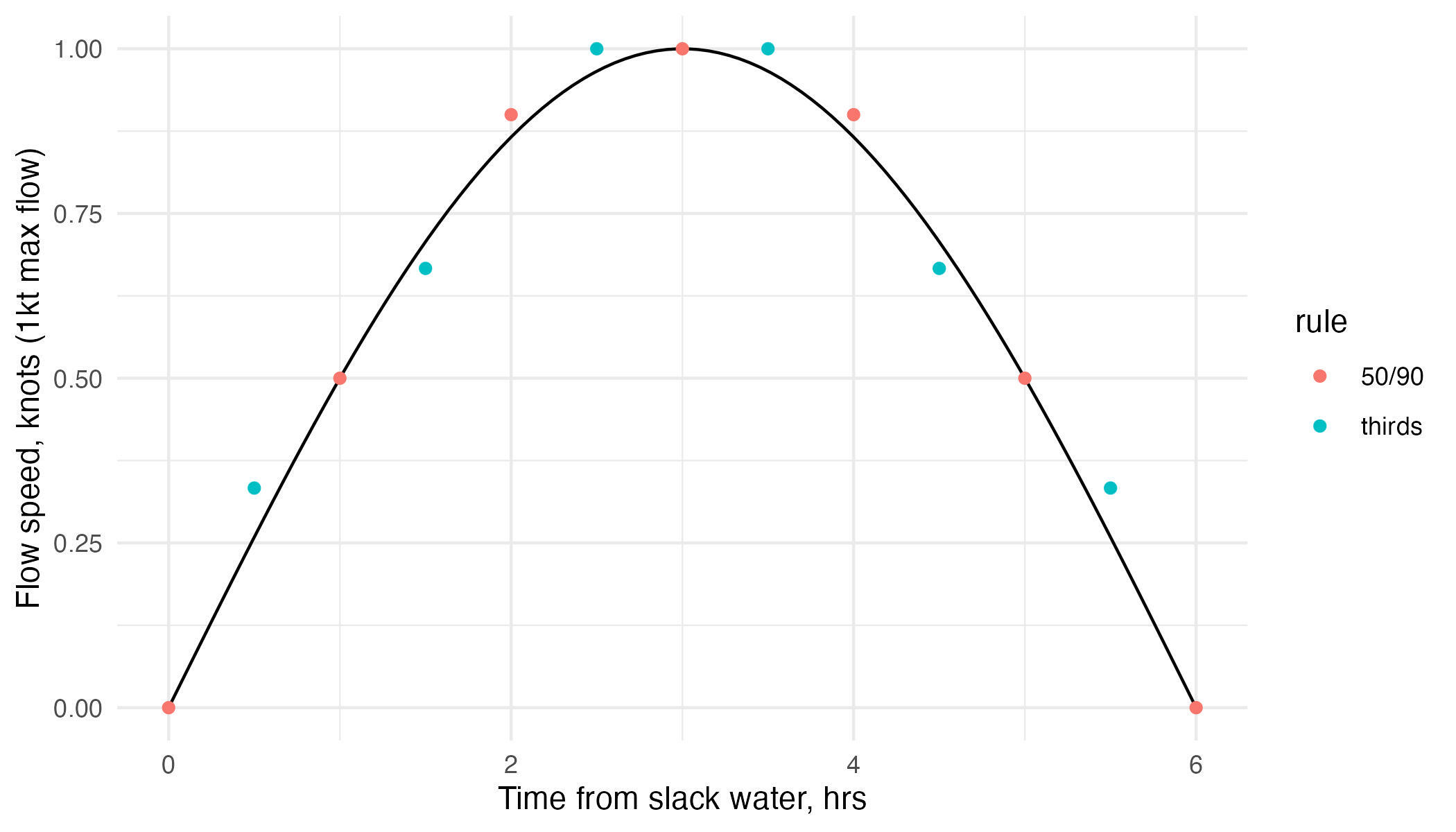
(Pedants will note that the rule of thirds actually refers to an average over the hour rather than a point estimate, but it's easier to plot this way, and the error is only ~1% or less).
As you can see, both rules provide pretty good approximations. Here's the numbers tabulated, with errors expressed as a proportion of the maximum flow speed:
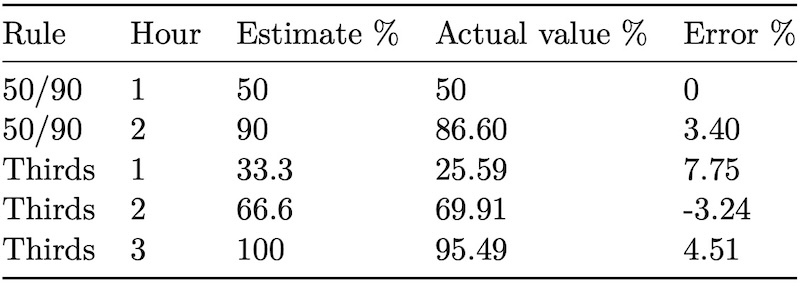
Again, the estimates are pretty good - all within 10% and most within 5%. If tidal flows follow nice sine waves, everything should be fine....
Real tidal flows
But is the assumption that tidal flows follow nice sine waves reasonable?
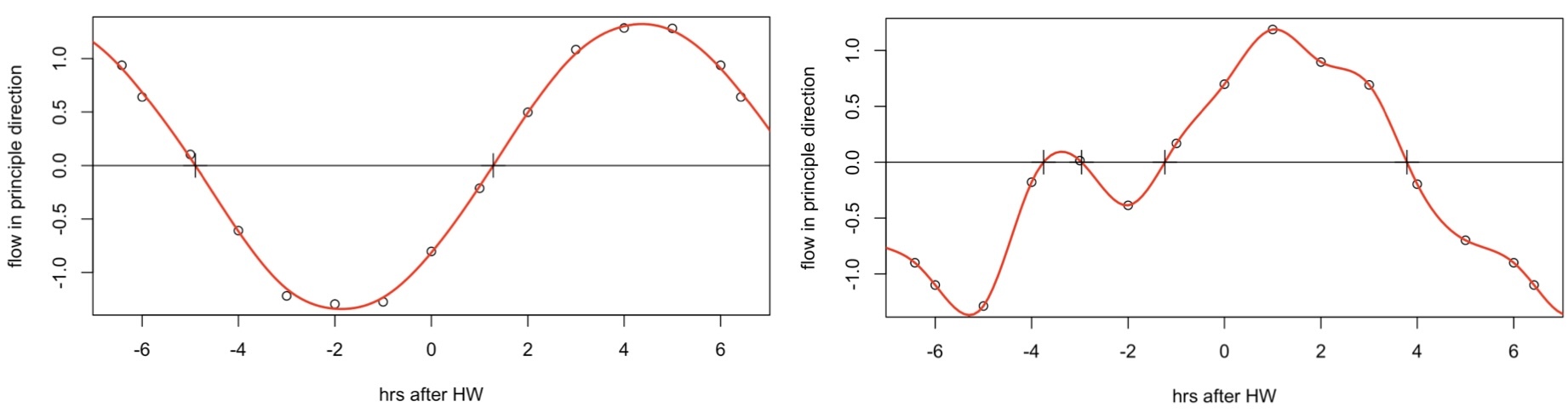
The left hand plot, taken the open ocean, far from land, appears to follow a nice smooth sine wave. The right hand plot, from under the short northern span of the Skye Bridge, clearly doesn't.
Can we do better than simply saying that the rules work well in some places, but not others? The plot below compares predictions using the 50/90 rule (fn) and the rule of thirds (th) to data from tidal diamonds.
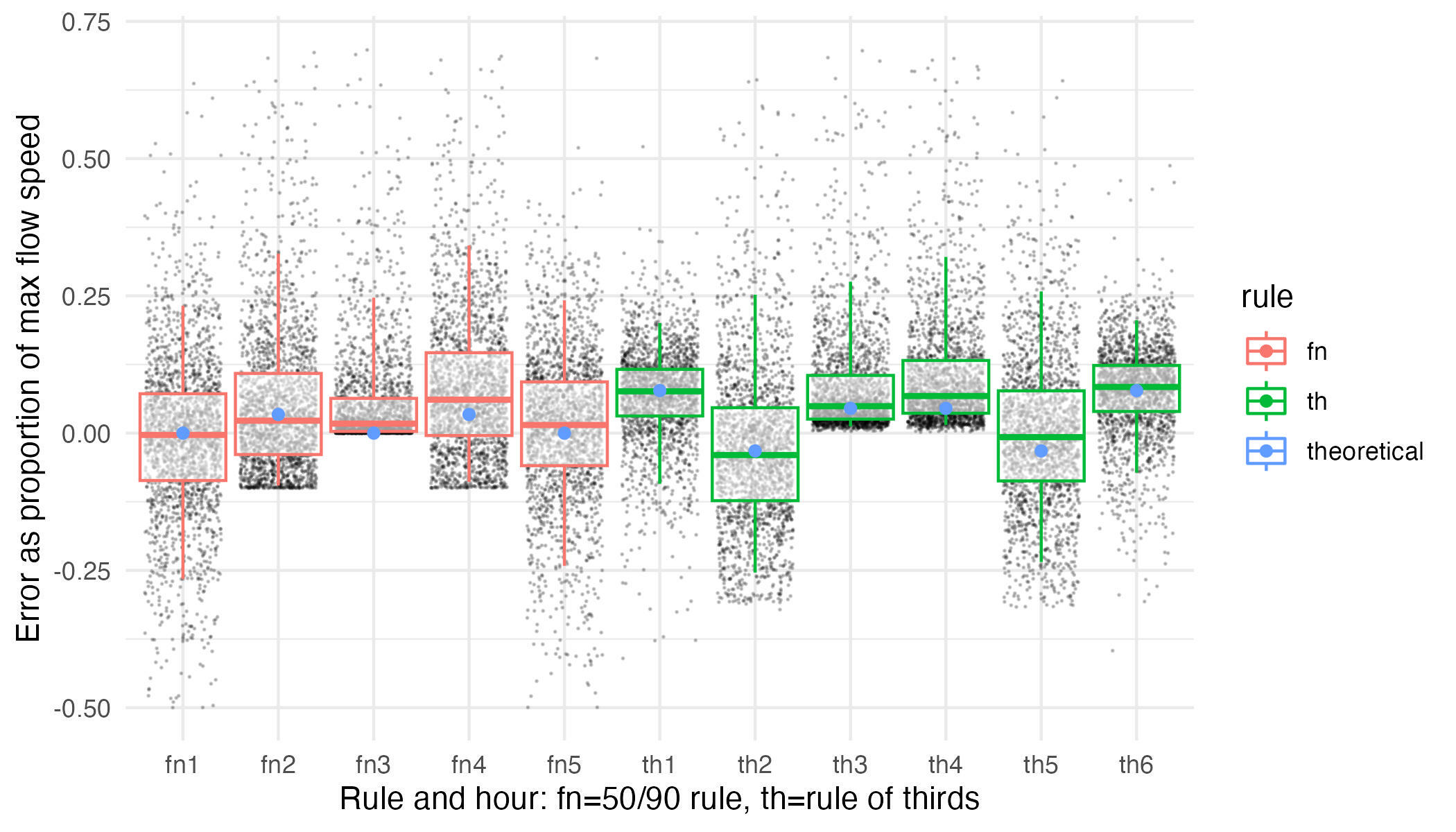
Each grey dot shows the error in one prediction for a specific hour of the tide. The boxes overlaid on top show where the ranges of 50% of errors fall. The lines extending from the boxes show the range for 90% of errors. Blue dots show the theoretical errors in approximating a sine wave that we considered above - we can see that the real data, on average, seems to correlate somewhat with the theory.
In general, the results are encouraging - despite all the weirdnesses of tidal flow, almost 90% of errors are less than 25-30% of maximum flow speed - i.e. less than a knot for a 3kt tidal stream. The errors are biased positive - i.e. you'll tend to over-estimate flow speeds rather than under-estimate them, which is reassuring.
Notice that for many of the hours, it's hard to under-estimate. This makes sense - if you're predicting that flow is 90% or 100% of the maximum flow in the cycle, you're unlikely to under-estimate. For the hours where this isn't the case, the errors are more symmetric, and typical plus or minus 25% of maximum flow speed, in 90% of cases. The first/last hour in the rule of thirds is more accurate - that's probably because flow speeds are lower in this part of the cycle, so errors measured as a proportion of maximum flow speed will be small.
Finally, looking at the 2nd/4th hour of the 50/90 rule and the 2nd/5th hour of the rule of thirds, there's a subtle indication that, on average, tidal flow curves are left-skewed - i.e. faster than expected flows occur earlier in the cycle, and slower flows later. See this post for a possible explanation.
We should be cautious of these conclusions. Tidal diamonds tend to be positioned away from coastlines and tide races, in places where big ships navigate. In practise, the data used here likely miss lots of odd inshore flows - and it's clear that the errors can still be quite large in some cases.
So, what to do?
I'm pleasantly surprised by what I found looking at the data shown above. The 50/90 rule and the rule of thirds remain good rules of thumb for planning.
However, there are clearly significant departures from the tidy sine wave behaviour in a large number of cases, even for the deep water locations that tidal diamonds tend to cover. And all this has assumed that the underlying data on slack water times and maximum flow speeds is accurate...
As ever, we should use the best tools and information available to us, but expect to be surprised sometimes.
