
I'm very aware that I don't know a great deal about surf. I tend not to seek it out - whilst surfing in a sea kayak is a good skill to have, I don't especially enjoy it, compared to, say, surfing a fast river wave in a planing hull boat. However, there's certainly a lot to be learned in the surf and I could really do with becoming more adept at finding friendly breaks. Anyhow - any hardcore surfers probably won't find what follows terribly insightful...
Like most sea kayakers, I'm aware that the period of a wave can be as important as its height. The period - the time between successive wave crests passing - provides a measure of the horizontal length of a wave, in the same wave that the height provides the vertical dimension. Actually, the length of the wave is simply 1.56 times the period in seconds squared, so the horizontal length increases rapidly with longer period.
The simple explanation normally given for the increased power of a long period wave is that the amount of water in the wave is very much greater. Whilst there's probably some truth in this for waves breaking on rocky shorelines, there's a problem with this explanation - it's the wave that moves, not the water within it.
The other important characteristic of long period waves that is sometimes overlooked is that they move faster than short period waves - the speed is simply 1.56 times the period. And it seems to be the increased speed at which the energy of ocean waves move that causes longer period swell to generate bigger surf waves.
The energy of a deep water ocean swell moves at a speed proportional to the period. But in shallow water, the wave energy moves at a speed determined only by the water depth. This means that the energy from long period swells gets compressed in shallow water - resulting in bigger waves.
A lot of different scientists seem to have looked at the question of how big surf waves get, but a simple and surprisingly accurate relationship was proposed by Paul Komar and Micheal Gaughan in 1972. Interestingly, Gaughan was an accomplished surfer, paddle boarder and ocean lifeguard.
Komar and Gaughan proposed that the surf height is 0.62 times the swell height to the power 0.8 times the period to the power 0.4. To save on calculator use on the beach, here's a plot of surf height against swell height and period:
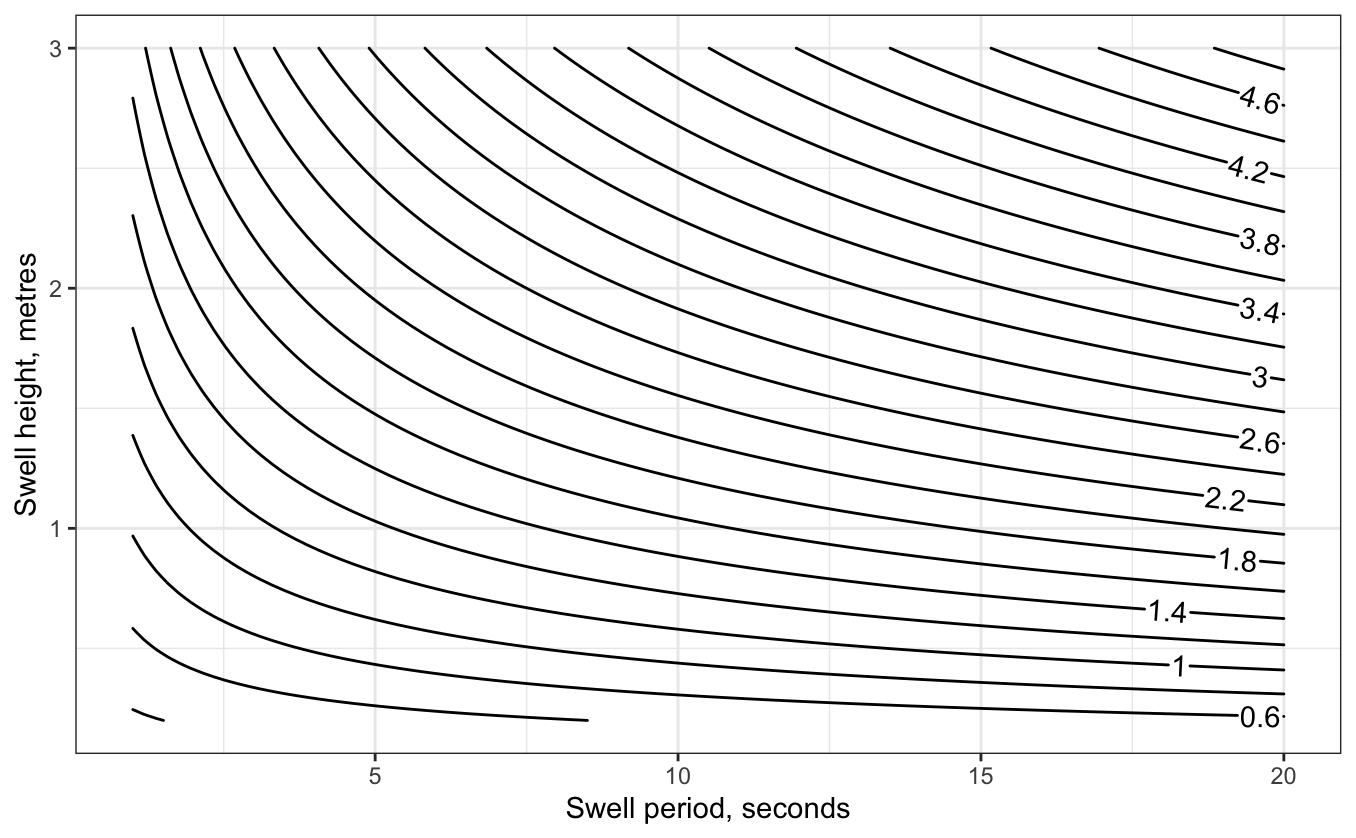
Perhaps more interesting is the degree to which the height of waves increases as they move into shallow water. Here's a plot showing the surf height as a multiple of swell height for a range of swell periods:
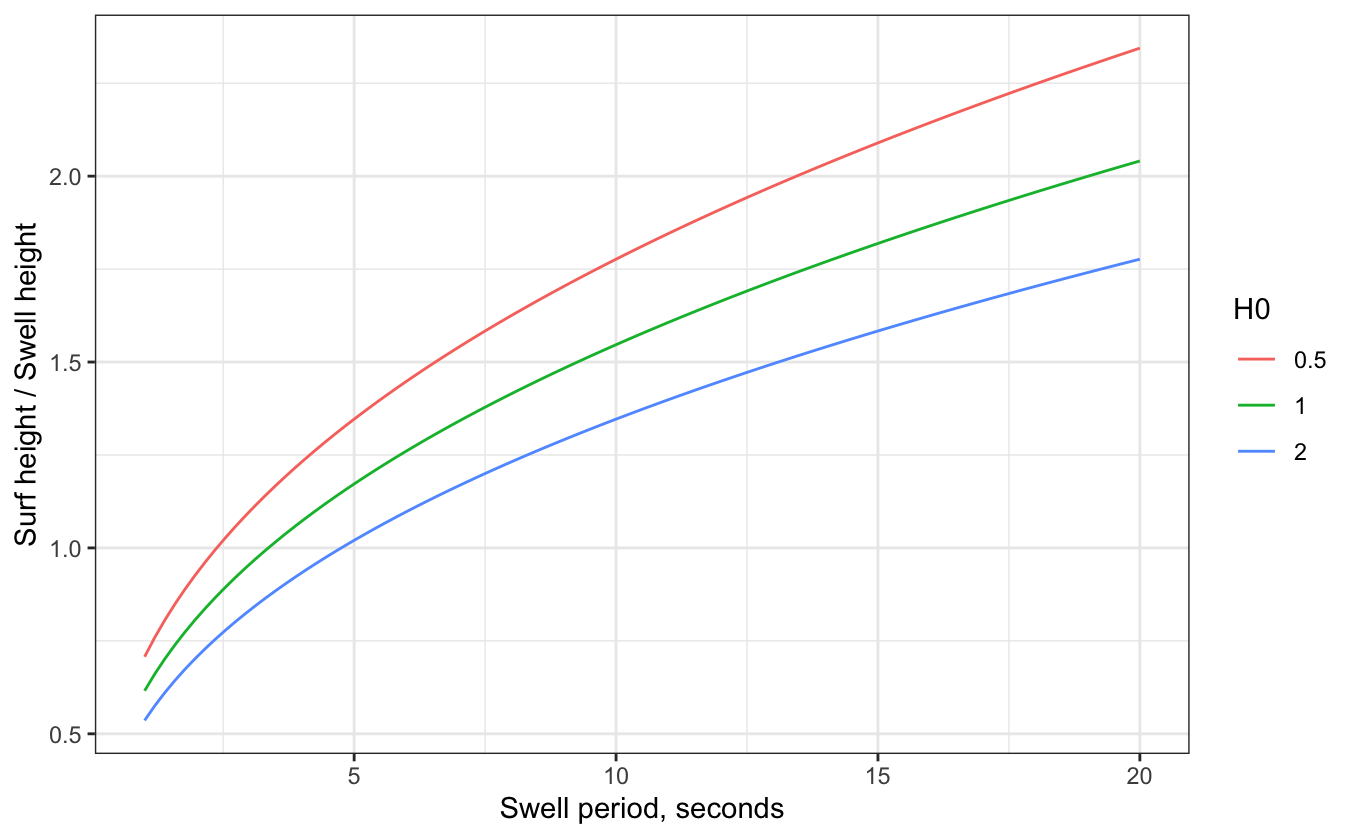
Behaviour varies a bit with swell height (H0 in the key). For 1 m waves of less than about 3 seconds period, the wave height decreases in shallow water, and the surf height is less than a metre. When the period increases to 10 seconds, surf height increases to 1.5 m. A 20 second period wave more than doubles in height, resulting in 2 m surf.
Clearly, this sort of simple relationship can only ever be an approximation - more complex relationships incorporate more variables, and all ignore the ways that waves get distorted and dispersed as they move into waves. But for those of us without a deep intuitive feel for surf forecasting, it's a handy rule of thumb.
